Question

Prove that: (sin x - 2 sin³ x)/(2 cos³ x - cos x) = tan x​

Answer 1

`\large\underline{\sf{Solution-}}`

We have to prove that:

`\rm\longmapsto ( \sin(x) - 2 \sin^(3) (x) )/(2 \cos^(3) (x) - \cos(x) ) = \tan(x)`

Taking LHS, we get:

`\rm = ( \sin(x) - 2 \sin^(3) (x) )/(2 \cos^(3) (x) - \cos(x) )`

We can take sin(x) as common from numerator part. We get:

`\rm = \frac{ \sin(x) \{1- 2 \sin^(2) (x) \}}{2 \cos^(3) (x) - \cos(x) }`

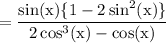
Similarly, we can take cos(x) as common from first two terms. We get:

`\rm = \frac{ \sin(x) \{1- 2 \sin^(2) (x) \}}{ \cos(x) \{ 2 \cos^(2) (x) -1\}}`

`\rm = ( \sin(x) )/( \cos(x) ) \cdot \frac{\{1- 2 \sin^(2) (x) \}}{\{ 2 \cos^(2) (x) -1\}}`

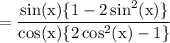
As we know that:

`\rm\longmapsto ( \sin(x) )/( \cos(x) ) = \tan(x)`

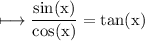
We get:

`\rm = \tan(x) \cdot \frac{\{1- 2 \sin^(2) (x) \}}{\{ 2 \cos^(2) (x) -1\}}`

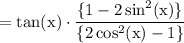
Now, we will expand the terms in fraction:

`\rm = \tan(x) \cdot \frac{\{1- \sin^(2) (x) - { \sin }^(2)(x) \}}{\{\cos^(2) (x) + \cos^(x)(x) -1\}}`

As we know that:

`\rm \longmapsto \sin^(2)(x)+cos^(2)(x)=1`

`\rm \longmapsto\cos^(2)(x)=1-sin^(2)(x)`

`\rm \longmapsto\cos^(2)(x) - 1=-sin^(2)(x)`

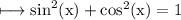
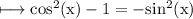
Now substitute the values in the expression, we get:

`\rm = \tan(x)\cdot \frac{ \cos^(2) (x)-{ \sin }^(2)(x)}{\cos^(2) (x) - \sin^(2) (x) }`

`\rm = \tan(x)\cdot1`

`\rm = \tan(x)`

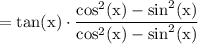
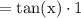
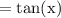
Here, we notice that LHS = RHS

Proved.