Answer:
0.01 (nearest hundredth)
Explanation:
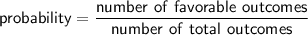
Total number of marbles (total outcomes) = 2 + 6 + 7 = 15
⇒ Probability of the first marble being red =
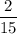
As there is no replacement, the total number marbles is now 15 and there is only 1 red marble left.
⇒ Probability of the second marble being red =
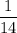
So the probability of the 1st and 2nd marble being red is:
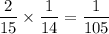 = 0.01 (nearest hundredth)
= 0.01 (nearest hundredth)