9514 1404 393
Answer:
(x, y, P) = (4/3, 8/3, 112/3)
Explanation:
In standard form, the constraints are all "less than or equal to", the variables are all non-negative, and the objective is maximization. This problem is presented in standard form.
4x +y ≤ 8
x +4y ≤ 12
x ≥ 0; y ≥ 0
maximize P = 6x +11y
__
Now we add the slack variables to make the constraints be "equal to". These have a coefficient of +1, and they, too, are non-negative. Here, we'll use s and t as the slack variables.
4x +y +s = 8
x +4y +t = 12
These equations, together with the objective function, are combined in a matrix-like "tableau" in which each row is the set of coefficients in one of the equations. Here, the columns represent x, y, s, t, P and the constants. The equation for the objective function is negated so we're trying to minimize that, as well.
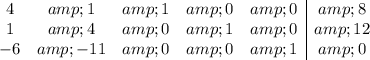
Find the column with the most negative value on the bottom row. (Column 2) Divide the rightmost column by this column and find the smallest quotient. Here, the quotients are 8/1 = 8, and 12/4 = 3. The smallest is 3. This identifies the 4 in column 2, row 2 as the "pivot."
Now, row operations are performed to make the pivot be 1 and other values in the pivot column be zero. This gives a new tableau. For example, the new values in row 1 are ...
4 -1/4, 1 -4/4, 1 -0/4, 0 -1/4, 0 -0/4, 8 -12/4
The new tableau is ...
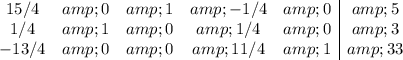
Now, the most negative number on the bottom row is in column 1, and the smallest quotient of column 6 and column 1 is in row 1. This makes 15/4 the "pivot". After the required row operations, the next tableau is ...
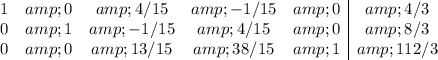
All values in the last row are non-negative, so we are finished. The rightmost column tells us the values of x, y, P that are the solution to the problem
(x, y, P) = (4/3, 8/3, 112/3)
__
The graphical solution confirms the Simplex solution.