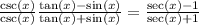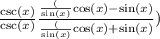Answer:
A∩B = 2,4
A-B = 1,3,5
sin (A+B) = sin(A)cos(B)+cos(A)sin(B)
Explanation:
A intersects U means the numbers that are coming to both A and U
set a minus set b of the numbers that are not common to B
multiply the numerator and the denominator by csc(x)
NOTE: I substituted x for theta as I don't have theta on my phone