Question

use the definition of countinuity to find the value of k so that the function is continuous for all real numbers

Answer 1

First of all, recall the definition of absolute value:

`|x| = \begin{cases}x&\text{if }x\ge0\\-x&\text{if }x<0\end{cases}`

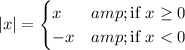
So if x < 4, then x - 4 < 0, so |x - 4| = -(x - 4), and the first case in h(x) reduces to

`(|x-4|)/(x-4)=(-(x-4))/(x-4) = -1`

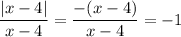
Next, in order for h(x) to be continuous at x = 4, the limits from either side of x = 4 must be equal and have the same value as h(x) at x = 4. From the given definition of h(x), we have

`h(4) = 5k-4\cdot4 = 5k-16`

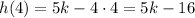
Compute the one-sided limits:

• From the left:

`\displaystyle \lim_(x\to4^-)h(x) = \lim_(x\to4)(|x-4|)/(x-4) = \lim_(x\to4)(-1) = -1`

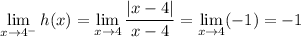
• From the right:

`\displaystyle \lim_(x\to4^+)h(x) = \lim_(x\to4)(5k-4x) = 5k-16`

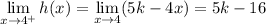
If the limits are to be equal, then

-1 = 5k - 16

Solve for k :

-1 = 5k - 16

15 = 5k

k = 3