Answer:
3²
Explanation:
You can rewrite the given problem as follows:
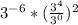
The first thing you must do is work on the terms inside the parenthesis.
According to the Zero exponent rule:
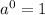 . This means that any number or variable raised to the 0 power will equal 1. Therefore, the denominator inside the parenthesis is 1.
. This means that any number or variable raised to the 0 power will equal 1. Therefore, the denominator inside the parenthesis is 1.
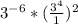

Next, according to the Power-to-Power Rule:
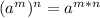
Therefore, you can multiply the exponent, 2, into
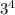 :
:
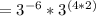
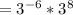
Then, the you can also apply the Negative Exponent Rule for rewriting
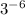 :
:
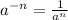
Therefore,
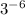 will become:
will become:
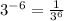
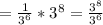
Finally, the Quotient Rule states that:

Therefore:
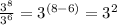
The correct answer is 3²