Question

Hey ! can anyone solve this ?​

Answer 1

(c) 1

Step-by-step explanation:

To solve such systems, "Lami's theorem" is used as it best relates the magnitudes of such coplanar, concurrent and non-collinear forces.

Statement:

When three forces acting at a point are in equilibrium, then each force is proportional to the sine of the angle between the other two forces.

In mathematical form:

`\boxed{ \mathsf{ (P)/( \sin( \theta _(1)) ) = (Q)/(\sin( \theta _(2)) ) = (R)/(\sin( \theta _(3)) ) }}`

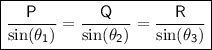
Solution:

According to the FBD, The given three forces are coplanar, concurrent(act at a same point), and in equilibrium.

Instead of θ₃, we have 150 and the value of sin(θ₁) is known.

Using Lami's :

`\implies \: \mathsf{ (R)/( \sin(150) ) = (P)/( \sin( \theta _(1) ) ) }`

• sin (150) = sin(180- 30)

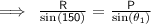
= sin 30

= 1/ 2

• P = 1.9318
• sin(θ₁) = 0.9659

`\implies \: \mathsf{ (R)/( (1)/(2) ) = (1.9318)/( 0.9659 ) }`

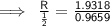
• R is multiplied by the reciprocal of ½ that is 2,
• upon solving the Right Hand Side, we get 2

`\implies \: \mathsf{ (2R)/(1 ) = (2)/( 1 ) }`

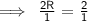
• Canceling 2 from both side

`\implies \mathsf{R \: = 1}`

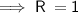
that is option C.