Answer:
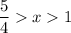

Explanation:
we would like to solve the following rational inequality
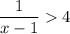
Note that we really CANNOT multiply both sides by x-1 as it can either be negative or positive however there're two methods of addressing this problem. Methods are as follows
Method-1:
In this method we would guess the answer by examining several values of x. Before we do so, we need to rearrange the inequality.
firstly, cancel 4 from both sides:

simplify:

now we can examine different values of x to test where -4x+5/x-1 is greater than 0.
At x = -1, -4x+5/x-1 is less than 0
At x = 0, -4x+5/x-1 is less than 0
At x = 1 , -4x+5/x-1 is undefined
At x = 5/4 ,-4x+5/x-1 is equal to 0
At x = 2 , -4x+5/x-1 is less than 0
It tells us the image that
The inequality is true on the interval (1,5/4)
Method-2:
In this method, we would consider nothing but algebra to solve the inequality. Likewise method-1, we need to rearrange the inequality. As I've already shown how to rearrange the inequality, I am skipping the steps for now. so rearranging the inequality yields
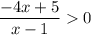
owing to algebra, we know that
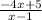 would be greater than 0 in case
would be greater than 0 in case
- Both the numerator and denominator is greater than 0
- Both the numerator and denominator is less than 0
thus it can be separated in two conditions
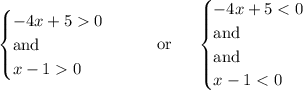
solve the inequalities:
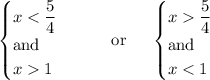
solve the "and" inequality or find the interception:

solve the "or" inequality or work out the union:
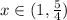
and we're done!