S O L U T I O N:
Let's assume that the given rectangle be ABCD and in that let ∆ABC be the right angled triangle formed from it.
Now we've been asked to find out the breadth. By applying Pythagoras theorem here in our rectangle ABCD we have,
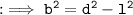
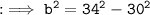
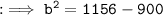


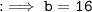
For the formula of area (a) = ? it is given by,
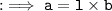
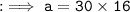
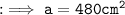
For the formula of perimeter (p) = ? is given by,



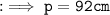
- The breadth, area and perimeter of rectangle is 16, 480cm² and 92cm.