Answer:
-7
Explanation:
We are given the following sequence:
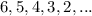
Checking if the sequence is arithmetic by using the following formula:
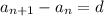
where d is a common difference. Common Difference means that these sequences must have same difference.
Let's check!
5-6 = -1
4-5 = -1
3-4 = -1
2-3 = -1
Since they are the same, the sequence is arithmetic.
General Term of Arithmetic Sequence
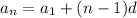
We know that a1 is 6 since 6 is the first term.
d is -1.
Our goal is to find a14. Therefore,
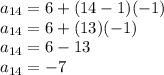
Therefore, the 14th term of sequence is -7.