Solve for x using logarithm: 25^x-3(5^x)=0

Question

asked Jun 13, 2023 197k views

1 Answer

MrGrigg · Answer 1 · 2023-06-17T05:13:14+0000

Answer:

x = 0.68

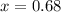
Explanation:

We would like to find out the value of x using logarithms of the given equation .The equation is ,

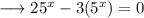
$\longrightarrow 25^x - 3(5^x)=0\\$

Add
3(5^x) on both sides,

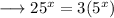
$\longrightarrow 25^x = 3(5^x)$

Using log to the base 10 on both sides, we have;

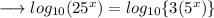
$\longrightarrow log_(10)(25^x) = log_(10)\{3(5^x)\}$

Recall that
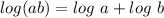$log(ab ) = log\ a + log\ b$ .

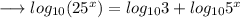
$\longrightarrow log_(10)(25^x)=log_(10)3 + log_(10)5^x$

Recall the properties of logarithm as
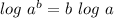$log\ a^b = b\ log\ a$ .

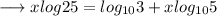
$\longrightarrow xlog25 = log_(10)3 + xlog_(10)5$

Again we can rewrite it as ,

$\longrightarrow xlog(5^2)=log_(10)3+xlog_(10)5\\$

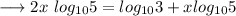
$\longrightarrow 2x\ log_(10)5 = log_(10)3+xlog_(10)5 \\$

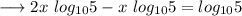
$\longrightarrow 2x\ log_(10)5-x\ log_(10)5 = log_(10)5$

Simplify,

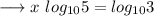
$\longrightarrow x\ log_(10)5=log_(10)3$

Divide both sides by log5 ,

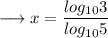
$\longrightarrow x =(log_(10)3)/(log_(10)5)$

Put on the values of log 3 and log5 ,

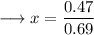
$\longrightarrow x =(0.47)/(0.69)$

Simplify,

$\longrightarrow \underline{{\underline{\boldsymbol{ x = 0.68}}}}$

And we are done!