Question

NO LINKS!!! Please help me with this problem. Part 10​

Answer 1

1053 feet

Explanation:

We choose to solve this problem using the tangent relation. It relates an acute angle to the adjacent and opposite legs of a right triangle.

Tan = Opposite/Adjacent

Opposite = Adjacent × Tan

__

For this to be useful here, we need to know the angles at the bottom of the figure. The left-side angle is 90° -69.2° = 20.8°. The right-side angle is 90°-65.5° = 24.5°. The tangent relation tells us ...

bridge left side + bridge right side = 880 ft

h×tan(20.8°) +h×tan(24.5°) = 880 ft

h = (880 ft)/(tan(20.8°) +tan(24.5°)) = (880 ft)/(0.37986 +0.45573)

h ≈ 1053.147 ft

The bridge is about 1053 feet high.

Answer 2

h = 1053 ft (to the nearest foot)

Explanation:

The sum of the interior angles of a triangle is 180°

Therefore, the angle at the bottom vertex of the triangle is:

180 - 69.2 - 65.5 = 45.3°

Sine rule:

`(a)/(\sin A)=(b)/(\sin B)=(c)/(\sin C)`

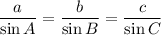
where A, B and C are the angles in a triangle, and a, b and c are the sides opposite those angles (e.g. side a is opposite angle A).

Now we can use the sine rule to calculate the length of the diagonal sides of the triangle.

Let a = the length of the right diagonal (the side with unknown length adjacent to angle 65.5°)

`(a)/(\sin(69.2))=(880)/(\sin(45.3))`

`\implies a=1157.353973...`

Now we can use the sine trig ratio to determine h:

`\sin(65.5)=(h)/(1157.353973...)`

`\implies h=1053.147292...`

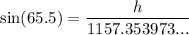
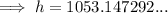
Therefore, h = 1053 ft (to the nearest foot)