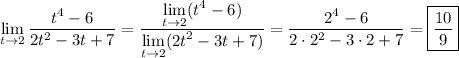I think you meant to say
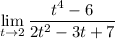
(as opposed to x approaching 2)
Since both the numerator and denominator are continuous at t = 2, the limit of the ratio is equal to a ratio of limits. In other words, the limit operator distributes over the quotient:
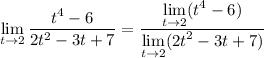
Because these expressions are continuous at t = 2, we can compute the limits by evaluating the limands directly at 2: