Question

1.) Find the length of the arc of the graph x^4 = y^6 from x = 1 to x = 8.

Answer 1

First, rewrite the equation so that y is a function of x :

`x^4 = y^6 \implies \left(x^4\right)^(1/6) = \left(y^6\right)^(1/6) \implies x^(4/6) = y^(6/6) \implies y = x^(2/3)`

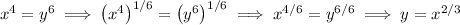
(If you were to plot the actual curve, you would have both
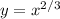`y=x^(2/3)` and
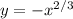`y=-x^(2/3)`, but one curve is a reflection of the other, so the arc length for 1 ≤ x ≤ 8 would be the same on both curves. It doesn't matter which "half-curve" you choose to work with.)

The arc length is then given by the definite integral,

`\displaystyle \int_1^8 \sqrt{1 + \left((\mathrm dy)/(\mathrm dx)\right)^2}\,\mathrm dx`

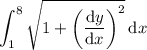
We have

`y = x^(2/3) \implies (\mathrm dy)/(\mathrm dx) = \frac23x^(-1/3) \implies \left((\mathrm dy)/(\mathrm dx)\right)^2 = \frac49x^(-2/3)`

Then in the integral,

`\displaystyle \int_1^8 \sqrt{1 + \frac49x^(-2/3)}\,\mathrm dx = \int_1^8 \sqrt{\frac49x^(-2/3)}\sqrt{\frac94x^(2/3)+1}\,\mathrm dx = \int_1^8 \frac23x^(-1/3) \sqrt{\frac94x^(2/3)+1}\,\mathrm dx`

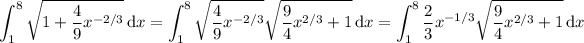
Substitute

`u = \frac94x^(2/3)+1 \text{ and } \mathrm du = (18)/(12)x^(-1/3)\,\mathrm dx = \frac32x^(-1/3)\,\mathrm dx`

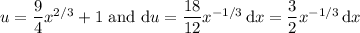
This transforms the integral to

`\displaystyle \frac49 \int_(13/4)^(10) √(u)\,\mathrm du`

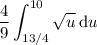
and computing it is trivial:

`\displaystyle \frac49 \int_(13/4)^(10) u^(1/2) \,\mathrm du = \frac49\cdot\frac23 u^(3/2)\bigg|_(13/4)^(10) = \frac8{27} \left(10^(3/2) - \left(\frac{13}4\right)^(3/2)\right)`

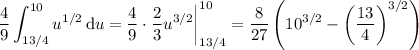
We can simplify this further to

`\displaystyle \frac8{27} \left(10√(10) - \frac{13√(13)}8\right) = \boxed{(80√(10)-13√(13))/(27)}`