Question

Find the coordinates of the vertex ​

Answer 1

You can either transform this quadratic equation into a vertex form which would rather painful or use derivatives.

I'll use dervatives. We know that vertex of a quadratic function is either its maxima or its minima, since the leading coefficient
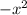`-x^2` has a negative prefix that means we get a downward turned parabola with minima being the vertex.

First we take the derivative with respect to x,

`(d)/(dx)-x^2-2x+3=-2x-2`

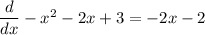
The derivative is esentially information what is the slope of a function at a particular x. When the slope is 0 we reached some sort of turning point, such as minima.

We therefore do,

`-2x-2=0\implies x=-1`

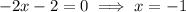
So at
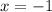`x=-1` there appears to be a minima or x-coordinate of the vertex of the function.

Plug the coordinate into the function to get y,

`y=f(-1)=-1+2+3=4`

So the vertex of the function is at
`\boxed{(-1,4)}`.

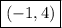Assuming you don't know derivatives, there is another way.

First compute the roots of the function,

`-x^2-2x+3=0`

`-(x-1)(x+3)=0`

`x_1=1,x_2=-3`

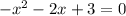
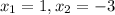
In the middle between
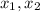`x_1,x_2` is an x coordinate of a vertex,

`x=(x_1+x_2)/(2)=(1-3)/(2)=-1`

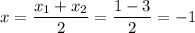
Just like we had before, we compute for y,
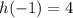`h(-1)=4` and again the result is
`\boxed{(-1,4)}`.

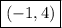Hope this helps :)