Answer:
see explanation
Explanation:
Given
6 - 4x - x²
Since the coefficient of the x² term < 0 then parabola has a maximum vertex.
To find the x- coordinate of the vertex
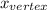 = -
= -
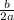
with a = - 1 and b = - 4 , then
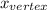 = -
= -
 = - 2
= - 2
The corresponding y- coordinate is found by substituting x = - 2 , that is
6 - 4(- 2) - (- 2)² = 6 + 8 - 4 = 10
vertex = (- 2, 10 )
The maximum value of the function is the y- coordinate of the vertex
Then maximum value is 10
Thus 6 - 4x - x² is never greater than 10