Answer:
D
Explanation:
We want to simplify the expression:
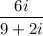
To do so, we can remove the imaginary unit in the denominator by multiply it by the conjugate.
The conjugate of a + bi is a - bi.
Hence, we will multiply the fraction by 9 - 2i:
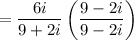
Multiply:
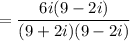
Difference of two squares:
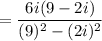
Simplify:
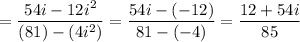
Hence, our answer is D.