Question

What is the midpoint of the segment that joins the points (-12, 12) and (-6, -1)?​

Answer 1

`\boxed {\boxed {\sf ( -9, (11)/(2)) }}`

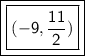
Explanation:

We are asked to find the midpoint of a segment. We essentially calculate the average of the x-coordinates and the y-coordinates using the following formula.

`( \frac {x_1+x_2}{2}, ( y_1 + y_2)/(2))`

In this formula, (x₁ , y₁) and (x₂ , y₂) are the endpoints of the segment. For this problem, the 2 endpoints are (-12, 12) and (-6, -1). If we match the variable and the corresponding value, we see that:

• x₁= -12
• y₁= 12
• x₂ = -6
• y₂ = -1

Substitute the values into the formula.

`( (-12 + -6)/(2), \frac{12 + -1} {2} )`

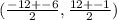
Solve the numerators.

• -12 + -6 = -12 -6 = -18
• 12 + -1 = 12-1 = 11

`( (-18)/(2), (11)/(2))`

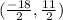
Divide.

`( -9, (11)/(2) )`

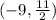
The midpoint of the segment is
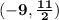`\bold {( -9, (11)/(2) )}`.