Question

Using the Factor Theorem, which of the polynomial functions has the zeros 2, radical 3 , and negative radical 3 ? f (x) = x3 – 2x2 – 3x + 6 f (x)= x3 – 2x2 + 3x + 6 f (x) = x3 + 2x2 – 3x + 6 f (x) = x3 + 2x2 – 3x – 6

Answer 1

A

`f(x) = x^3 - 2x^2 -3x + 6`

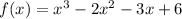
Explanation:

According to the Factor Theorem, if (x - k) is a factor of a polynomial P(x), then P(k) must equal zero.

We are given that a polynomial function has the zeros 2, √3, and -√3. So, we can let k = 2, √3, -√3.

So, according to the Factor Theorem, P(2), P(√3) and P(-√3) must equal 0.

Testing each choice, we can see that only A is true:

`\displaystyle f(x) = x^3 - 2x^2 - 3x + 6`

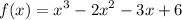
Testing all three values yields that:

`\displaystyle \begin{aligned} f(2) &= (2)^3 - 2(2)^2 -3(2) + 6 \\ &= (8) - (8) -(6) + (6) \\ &= 0\stackrel{\checkmark}{=}0 \\ \displaystyle f(√(3)) &= (√(3))^3 - 2(√(3))^2 - 3(√(3)) + 6 \\ &=(3√(3)) -(6)-(3√(3)) + 6 \\ &= 0\stackrel{\checkmark}{=}0 \\ f(-√(3)) &= (-√(3))^3 - 2(-√(3))^2 - 3(-√(3)) + 6 \\ &=(-3√(3)) -(6)+(3√(3)) + 6 \\ &= 0\stackrel{\checkmark}{=}0 \end{aligned}`

Hence, our answer is A.