Use the area of rectangle formula which is:
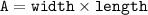
Given that:
- area of rectangle = 16
- width = 8
- length = x-5
Substitute in the formula:
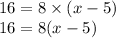
Distribute 8 in the expression:
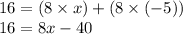
Move -40 to another side and change from -40 to 40.
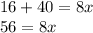
Then move 8 to divide 56, leaving only x.
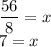
Hence, the answer is:
