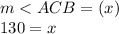Answer:

Explanation:
1. Approach
Refer to the attached diagram of the figure for further explanation. In this problem, one is asked to solve for the degree measure of arc (x). The easiest method to do so is to use the triangle (CAB). One can solve for the measure of angle (<CBA) by using the tangent to radius theorem. Then one can solve for the measure of angle (CAB) by using the base angles theorem. Then one can use the sum of angles in a triangle theorem to solve for angle (<BCA). Finally, one can use the central angles theorem to solve for the arc (x).
2. Find the measure of angles in the triangle
A. Find the measure of angle (<CBE)
As per the given image, lines (BE) and (AE) are tangent. This means that they intersect the circle at exactly one point. A radius is the distance from the center of a circle to the circumference or outer edge of a circle. All radii in a single circle are congruent. The radius of tangent theorem states that, when a tangent intersects a circle at a point of tangency, and a radius also intersects the point of tangency, the angle between the radius and the tangent is a right angle. One can apply this here by stating the following:
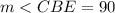
Express angle (<CBE) as the sum of two other angles:
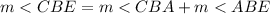
Substitute with the given and found information:
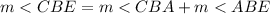
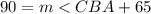
Inverse operations,
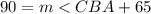

B. FInd the measure of angle (<CAB)
As stated above all radii in a single circle are congruent. This means that lines (CB) and (CA) are equal. Therefore, the triangle (CAB) is an isosceles triangle. One property of an isosceles triangle is the base angles theorem, this theorem states that the angles opposite the congruent sides of an isosceles triangle are congruent. Applying this theorem to the given problem, one can state the following:

C. Find the measure of angle (<ACB)
The sum of angles in any triangle is (180) degrees. One can apply this theorem here to the given triangle by adding up all of the angles and setting the result equal to (180) degrees. This is shown in the following equation:
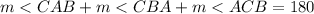
Substitute,
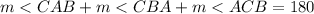
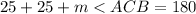
Simplify,
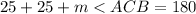
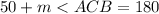
Inverse operations,
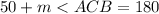
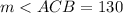
3. Find the measure of arc (x)
The central angles theorem states that when an angle has its vertex on the center of the circle, its angle measure is equivalent to the measure of the surrounding arc. Thus, one apply this theorem here by stating the following: