Problem 4.2
We're told that y = 2x is the equation of the tangent line through point Q.
Plug this into the equation of the circle (that was given at the very top of the page). Solve for x.
x^2 + y^2 - 18x - 6y + 45 = 0
x^2 + (2x)^2 - 18x - 6(2x) + 45 = 0 ... every "y" replaced with "2x"
x^2 + 4x^2 - 18x - 12x + 45 = 0
5x^2 - 30x + 45 = 0
5(x^2 - 6x + 9) = 0
5(x-3)^2 = 0
(x-3)^2 = 0
x-3 = 0
x = 3
This then means y = 2x = 2*3 = 6
The coordinates of point Q are (x,y) = (3,6)
----------
Answer: (3,6)
===========================================================
Problem 4.3
We first need to find the location of point S. That will involve solving this system of equations

which represent the equations of lines SR and QS in that exact order.
Like before, we'll apply substitution to solve for x.
x-2y+12 = 0
x-2(2x)+12 = 0
x-4x+12 = 0
-3x+12 = 0
12 = 3x
12/3 = x
4 = x
x = 4
Which leads to y = 2x = 2*4 = 8
Point S is located at (4,8)
Point R is given to be located at (6,9)
Apply the distance formula for these two points to find the length of SR (aka the distance from S to R).
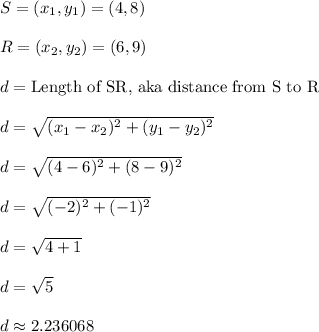
The exact length is
 and that approximates to roughly 2.236068 units.
and that approximates to roughly 2.236068 units.
----------
Answer:
Exact length =
 units
units
Approximate length = 2.236068 units