Question

Calculus!

The volume of a substance, A, measured in cubic centimeters increases according to the exponential growth model dA/dt = 0.3A, where t is measured in hours. The volume of another substance, B, also measured in cubic centimeters increases at a constant rate of 1 cm^3 per hour according to the linear model dB/dt = 1. At t = 0, substance A has a volume A(0) = 3 and substance B has size B(0) = 5. At what time will both substances have the same volume?

Would it be correct to write the growth model of substance B as x + 5? And how could I write the growth model of substance A? Thank you in advance, and sorry for the poor formatting.

Answer 1

The two substances will have the same volume after approximately 3.453 hours.

Explanation:

The volume of substance A (measured in cubic centimeters) increases at a rate represented by the equation:

`\displaystyle (dA)/(dt) = 0.3 A`

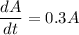
Where t is measured in hours.

And substance B is represented by the equation:

`\displaystyle (dB)/(dt) = 1`

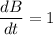
We are also given that at t = 0, A(0) = 3 and B(0) = 5.

And we want to find the time(s) t for which both A and B will have the same volume.

You are correct in that B(t) is indeed t + 5. The trick here is to multiply both sides by dt. This yields:

`\displaystyle dB = 1 dt`

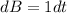
Now, we can take the integral of both sides:

`\displaystyle \int 1 \, dB = \int 1 \, dt`

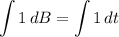
Integrate. Remember the constant of integration!

`\displaystyle B(t) = t + C`

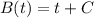
Since B(0) = 5:

`\displaystyle B(0) = 5 = (0) + C \Rightarrow C = 5`

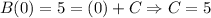
Hence:

`B(t) = t + 5`

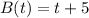
We can apply the same method to substance A. This yields:

`\displaystyle dA = 0.3A \, dt`

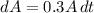
We will have to divide both sides by A:

`\displaystyle (1)/(A)\, dA = 0.3\, dt`

Now, we can take the integral of both sides:

`\displaystyle \int (1)/(A) \, dA = \int 0.3\, dt`

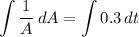
Integrate:

`\displaystyle \ln|A| = 0.3 t + C`

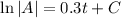
Raise both sides to e:

`\displaystyle e^(\ln |A|) = e^(0.3t + C)`

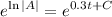
Simplify:

`\displaystyle |A| = e^(0.3t) \cdot e^C = Ce^(0.3t)`

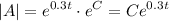
Note that since C is an arbitrary constant, e raised to C will also be an arbitrary constant.

By definition:

`\displaystyle A(t) = \pm C e^(0.3t) = Ce^(0.3t)`

Since A(0) = 3:

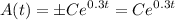
`\displaystyle A(0) = 3 = Ce^(0.3(0)) \Rightarrow C = 3`

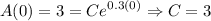
Therefore, the growth model of substance A is:

`A(t) = 3e^(0.3t)`

To find the time(s) for which both substances will have the same volume, we can set the two functions equal to each other:

`\displaystyle A(t) = B(t)`

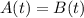
Substitute:

`\displaystyle 3e^(0.3t) = t + 5`

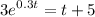
Using a graphing calculator, we can see that the intersect twice: at t ≈ -4.131 and again at t ≈ 3.453.

Since time cannot be negative, we can ignore the first solution.

In conclusion, the two substances will have the same volume after approximately 3.453 hours.