Question

Is the point (11,5) on the parabola? Show or explain your work.

Answer 1

The point (11, 5) is NOT on the parabola.

Explanation:

Let
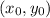`(x_0,y_0)` be any point on the parabola

Let
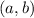`(a, b)` be the focus

Let
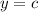`y=c` be the the directrix

Distance between
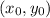`(x_0,y_0)` and the focus:
`√((x_0-a)^2+(y_0-b)^2)`

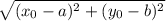
Distance between
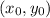`(x_0,y_0)` and the directrix is
`|y_0-c|`

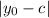
Given:

• focus (6, 4)
• directrix y = 0

Therefore:

Distance between
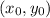`(x_0,y_0)` and the focus:
`√((x_0-6)^2+(y_0-4)^2)`

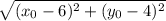
Distance between
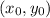`(x_0,y_0)` and the directrix is
`|y_0-0|`

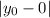
Equate the two expressions and solve for
`y_0`:

`√((x_0-6)^2+(y_0-4)^2)=|y_0-0|`

`\implies (x_0-6)^2+(y_0-4)^}=(y_0-0)^2`

`\implies {x_0}^2-12x_0+36+{y_0}^2-8y_0+16={y_0}^2`

`\implies {x_0}^2-12x_0-8y_0+52=0`

`\implies 8y_0={x_0}^2-12x_0+52`

`\implies y_0=\frac18{x_0}^2-(12)/(8)x_0+(52)/(8)`

`\implies y_0=\frac18{x_0}^2-\frac32x_0+(13)/(2)`

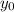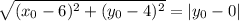
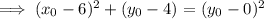
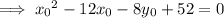
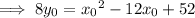
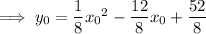
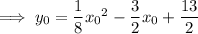
Now rewrite with (x, y):

`\implies y=\frac18x^2-\frac32x+(13)/(2)`

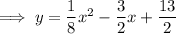
Therefore, this is the equation of the parabola

To determine if the point (11, 5) is on the parabola, input x = 11 into the equation:

`\implies y=\frac18(11)^2-\frac32(11)+(13)/(2)`

`\implies y=(41)/(8)`

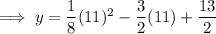
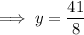
So the point (11, 5) is NOT on the parabola.