Question

A 26 foot ladder is lowered down a vertical wall at a rate of 3 feet per minute. The base of the ladder is sliding away from the wall. A. At what rate is the ladder sliding away from the wall when the base of the ladder is 10 feet from the wall

Answer 1

`7.2\:\text{ft/s}`

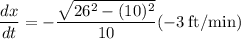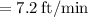
Explanation:

We can apply the Pythagorean theorem here:

`26^2 = x^2 + y^2\:\:\:\:\:\:\:\:\:(1)`

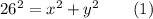
where x is the distance of the ladder base from the wall and y is the distance of the ladder top from the ground. Taking the time derivative of the expression above, we get

`0 = 2x(dx)/(dt) + 2y(dy)/(dt)`

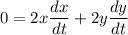
Solving for
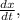`(dx)/(dt),` we get

`(dx)/(dt) = -(y)/(x)(dy)/(dt)`

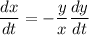
We can replace y by rearranging Eqn(1) such that

`y = √(26^2 - x^2)`

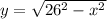
Therefore,

`(dx)/(dt) = - (√(26^2 - x^2))/(x)(dy)/(dt)`

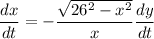
Since y is decreasing as the ladder is being lowered, we will assign a negative sign to
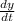`(dy)/(dt)`. Hence,

`(dx)/(dt) = - (√(26^2 - (10)^2))/(10)(-3\:\text{ft/min})`

`\:\:\:\:\:\:\:= 7.2\:\text{ft/min}`