Question

The volume of the donut created when the circle {x}^{2}+{y}^{2}=4 is rotated around the line x=4.

Answer 1

32π²

Explanation:

To solve this, we can use the washer method.

We can start by dividing the donut into horizontal sections. Each horizontal section is a hollow cylinder, with the inner cylinder's radius being the difference between x=4 and x²+y²=4, which can also be written as x = √(4-y²) (we do not need the negative x values as the inner cylinder does not encompass any part of the circle). This is (4-√(4-y²)) .

Similarly, the outer radius will be equal to the difference between 4 and the other side of the circle. This encompasses the circle and the extra area in between, so x will be negative here, making it (4-(-√(4-y²)) = 4+√(4-y²).

The area of the cylinder for a given Δy can be represented by

(4+√(4-y²))²πΔy - (4-√(4-y²))²πΔy. This space represents a cylinder in the donut created with the height being Δy. Simplifying the equation, we get

(4+√(4-y²))²πΔy - (4-√(4-y²))²πΔy = πΔy((4+√(4-y²))²- (4-√(4-y²))²)

(4+√(4-y²))²- (4-√(4-y²))² = 16+8√(4-y²) + 4-y² - (16-8√(4-y²)+4-y²)

= 16√(4-y²)

(4+√(4-y²))²πΔy - (4-√(4-y²))²πΔy = 16√(4-y²)πΔy

The donut ranges from all y values from -2 to 2, and to add all the areas of the cylinders up between these y values, we can make this an integral,

`\int\limits^2_(-2) {16\pi √(4-y^2) \, dy\\`

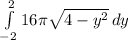
perform u substitution, make y = 2sin(u). If y=2sin(u), then y/2 = sin(u) and arcsin(y/2) = u

`\int\limits^2_(-2) {16\pi* 2cos(u) √(4-4sin^2(u)) \, du\\`

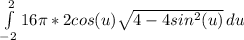
take the 32π out of the integral

`32\pi\int\limits^2_(-2) {cos(u) √(4-4sin^2(u)) \, du\\\\= 32\pi\int\limits^2_(-2) {cos(u) *2cos(u)} \, du\\\\\\= 32\pi\int\limits^2_(-2) {2cos&^2(u)} \, du\\\\\\\\\\`

take the 2 out of the integral

`64\pi\int\limits^2_(-2) {cos&^2(u)} \, du\\`

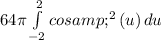
One reduction formula we can use here is that

`\int\limits {cos^nx} \, dx = (1)/(n) cos^(n-1)(x) sin(x) +(n-1)/(n) \int\limits {cos^(n-2) (x)} \, dx`

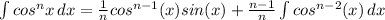
Applying that here, we get

`\int\limits {cos^2u} \, du = 0.5cos(u)sin(u) + 0.5 \int\limits {1} \, du\\= 0.5cos(arcsin(y/2))sin(arcsin(y/2)) + 0.5(arcsin(y/2))`

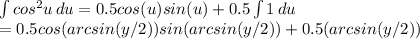
take that cos(arcsin(x)) = √(1-x²) and sin(arcsin(x)) = x and we get

`\int\limits {cos^2u} \, du = 0.5√(1-y^2/4)*(y/2) + 0.5arcsin(y/2)\\= 0.25y√(1-y^2/4) + 0.5arcsin(y/2)\\`

multiply this back with the 64π to get

`16\pi y√(1-y^2/4) + 32\pi arcsin(y/2)`

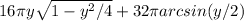
as our integral. Applying this to the bounds of [-2, 2], we get

16π(2)√(1-4/4) + 32πarcsin(1) - (16π(-2)√(1-4/4) + 32πarcsin(-1))

= 0 + 32π(π/2) - 32π(-π/2)

= 16π²+16π²

= 32π²

as our answer