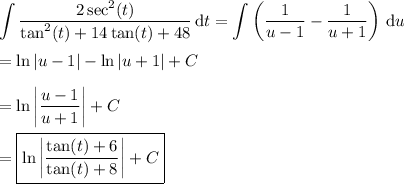Question

Make a substitution to express the integrand as a rational function and then evaluate the integral. (Remember to use absolute values where appropriate. Use C for the constant of integration.)

2 sec^2(t)/tan^2(t) + 14 tan(t) + 48 dt

Answer 1

Complete the square in the denominator to get

tan²(t ) + 14 tan(t ) + 48 = tan²(t ) + 14 tan(t ) + 49 - 1

… = (tan(t ) + 7)² - 1

Substitute u = tan(t ) + 7 and du = sec²(t ) dt. Then the integral becomes

`\displaystyle \int (2\sec^2(t))/(\tan^2(t)+14\tan(t)+48) \,\mathrm dt = 2 \int (\mathrm du)/(u^2-1)`

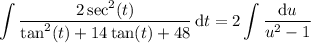
Separate the integrand into partial fractions:

`\frac1{u^2-1} = \frac12 \left(\frac1{u-1}-\frac1{u+1}\right)`

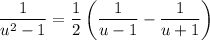
Then we get

`\displaystyle \int (2\sec^2(t))/(\tan^2(t)+14\tan(t)+48) \,\mathrm dt =  \int \left(\frac1{u-1}-\frac1{u+1}\right)\,\mathrm du \\\\ =\ln|u-1|-\ln|u+1| + C \\\\ = \ln\left|(u-1)/(u+1)\right|+C \\\\ = \boxed+C`