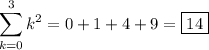Answer:
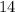
Explanation:
Given

Let's break down each part. The input at the bottom, in this case
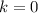 , is assigning an index
, is assigning an index
 at a value of
at a value of
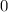 . This is the value we should start with when substituting into our equation.
. This is the value we should start with when substituting into our equation.
The number at the top, in this case 3, indicates the index we should stop at, inclusive (meaning we finish substituting that index and then stop). The equation on the right, in this case
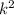 , is the equation we will substitute each value in. After we substitute our starting index, we'll continue substituting indexes until we reach the last index, then add up each of the outputs produced.
, is the equation we will substitute each value in. After we substitute our starting index, we'll continue substituting indexes until we reach the last index, then add up each of the outputs produced.
Since
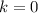 is our starting index, start by substituting this into
is our starting index, start by substituting this into
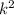 :
:

Now continue with
 :
:

Repeat until we get to the ending index,
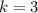 . Remember to still use
. Remember to still use
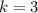 before stopping!
before stopping!
Substituting
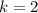 :
:
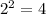
Substituting
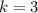 :
:
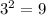
Since 3 is the index we end at, we stop here. Now we will add up each of the outputs:
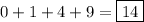
Therefore, our answer is: