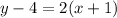Answer:
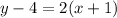
Explanation:
Point-slope form is equal to

where y and y1 are the known y coordinates of two points on the line, and x and x1 are the known x coordinates of two points on the line. All we need now is m, which is the slope:

We can simplify negative one minus negative two as positive 1.
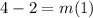
4 minus 2 is 2, so m times 1 is 2. That means m is 2.
Now, we have the slope, so we can convert to point-slope form using one of the two points. Let's use (-1, 4). We can plug those values in for x1 and y1: