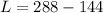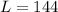Question

You have 576 feet of fencing to enclose a rectangular plot of land. Find the dimensions of the rectangular plot that would maximize the area. List the smaller number first.

Answer 1

The dimension that maximizes area is 144ft by 144ft

Explanation:

Given

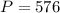`P = 576` -- perimeter

Required

The dimension that gives maximum area

Perimeter is calculated as:

`P= 2 * (L + W)`

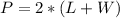
So, we have:

`2 * (L + W) = 576`

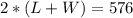
Divide through by 2

`L + W = 288`

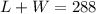
Make L the subject

`L = 288 -W`

Area is calculated as:

`A = L * W`

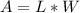
Substitute
`L = 288 -W`

`A = (288 - W) * W`

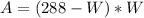
Open bracket

`A = 288W - W^2`

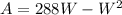
Differentiate A with respect to W

`A' = 288 - 2W`

Set to 0 to calculate W

`288 - 2W = 0`

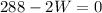
Collect like terms

`2W = 288`

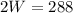
Divide by 2

`W = 144`

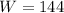
Recall that:

`L = 288 -W`

`L = 288 - 144`

`L = 144`