Answer:
i) The period of the particle is 0.3 seconds
ii) The angular velocity is approximately 20.94 rad/s
iii) The linear velocity is approximately 1.047 m/s
iv) The centripetal acceleration is approximately 6.98 m/s²
Step-by-step explanation:
The given parameters are;
The number of revolution of the particle, n = 800 revolution
The time it takes the particle to make 800 revolutions = 4 minutes
The dimension of the circle = 5 cm = 0.05 m
Given that the dimension of the circle is the radius of the circle, we have;
i) The period of the particle, T = The time to complete one revolution
T = 1/(The number of revolutions per second)
∴ T = 1/(800 rev/(4 min × 60 s/min)) = 3/10 s
The period, T = 3/10 seconds = 0.3 seconds
ii) The angular velocity, ω = Angle covered/(Time)
800 revolutions in 4 minutes = Angle of (800 × 2·π) in 4 minutes
∴ ω = (800 × 2·π)/(4 × 60) = 20·π/3
The angular velocity, ω = 20·π/3 rad/s ≈ 20.94 rad/s
iii) The linear velocity, v = r × ω
∴ The linear velocity, v = 0.05 m × 20·π/3 rad/s = π/3 m/s ≈ 1.047 m/s
iv) The centripetal acceleration,
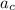 = v²/r
= v²/r
∴ The centripetal acceleration,
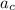 = (π/3)²/(0.05) = 20·π/9
= (π/3)²/(0.05) = 20·π/9
The centripetal acceleration,
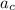 = 20·π/9 m/s² ≈ 6.98 m/s²
= 20·π/9 m/s² ≈ 6.98 m/s²