Question

Seeds are often treated with fungicides to protect them in poor-draining, wet environments. A small-scale trial, involving six treated and six untreated seeds, was conducted prior to a large-scale experiment to explore how much fungicide to apply. The seeds were planted in wet soil, and the number of emerging plants were counted. If the solution was not effective and five plants actually sprouted.

Required:
What is the probability that all five plants emerged from treated seeds?

Answer 1

0.0076 = 0.76% probability that all five plants emerged from treated seeds

Explanation:

The plants were chosen without replacement, which means that the hypergeometric distribution is used to solve this question.

Hypergeometric distribution:

The probability of x successes is given by the following formula:

`P(X = x) = h(x,N,n,k) = (C_(k,x)*C_(N-k,n-x))/(C_(N,n))`

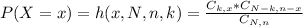
In which:

x is the number of successes.

N is the size of the population.

n is the size of the sample.

k is the total number of desired outcomes.

Combinations formula:

`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

In this question:

6 + 6 = 12 seeds, which means that
`N = 12`

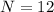
6 treated, which means that
`k = 6`

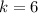
Five sprouted, which means that
`n = 5`

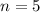
What is the probability that all five plants emerged from treated seeds?

This is P(X = 5). So

`P(X = x) = h(x,N,n,k) = (C_(k,x)*C_(N-k,n-x))/(C_(N,n))`

`P(X = 5) = h(5,12,5,6) = (C_(6,5)*C_(6,0))/(C_(12,5)) = 0.0076`

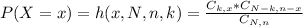
0.0076 = 0.76% probability that all five plants emerged from treated seeds