Question

CHEGG Over the course of a multi-stage 4820-km bicycle race, the front wheel of an athlete's bicycle makes 2.40x106 revolutions. How many revolutions would the wheel have made during the race if its radius had been 1.4 cm larger?

Answer 1

θ' = 14.44 ×
`10^(6)`

Step-by-step explanation:

given data

total distance is d = 4820

radius = 1.4 cm

solution

we get here total angle by which the wheel rotates traveling is express as

⇒
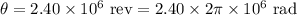`\theta=2.40*10^6\ \rm{rev}=2.40* 2\pi*10^6\ \rm{rad}` ................1

and

total angle (θ) and the total distance (d) express as

⇒ d = r × θ ...............2

here r is radius

and here rotated through some other angle θ' so put value in given equation and find revolutions

⇒ d = (r+r)θ' ........3

here r = d/θ

so

⇒
`d = ( (d)/(\theta)+r) \theta'`

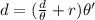
so put value and get θ'

⇒ θ' = 2.40 × 2π ×
`10^(6)` ×
`(4820 * 10^3)/(4820 * 10^3 +0.014 * 2.40 * 2 * \pi * 10^6)`

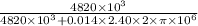
⇒ θ' = 14.44 ×
`10^(6)` rev