Question

An adult can lose or gain two pounds of water ina course of a day. Assume that the changes in water weight isuniformly distributed between minus two and plus two pounds in aday. What is the standard deviation of your weight over a day?

Answer 1

The standard deviation of your weight over a day is of 1.1547 pounds.

Explanation:

Uniform probability distribution:

An uniform distribution has two bounds, a and b, and the standard deviation is:

`S = \sqrt{((b-a)^2)/(12)}`

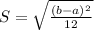
Assume that the changes in water weight is uniformly distributed between minus two and plus two pounds in a day.

This means that
`a = -2, b = 2`

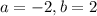
What is the standard deviation of your weight over a day?

`S = \sqrt{((2 - (-2))^2)/(12)} = \sqrt{(4^2)/(12)} = \sqrt{(16)/(12)} = 1.1547`

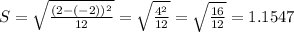
The standard deviation of your weight over a day is of 1.1547 pounds.