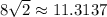Question

Please help!!

Find BD​

Answer 1

Answer:
`8√(2)`

==========================================================

Work Shown:

Focus entirely on triangle ABD (or on triangle BCD; both are identical)

The two legs of this triangle are AB = 8 and AD = 8. The hypotenuse is unknown, so we'll say BD = x.

Apply the pythagorean theorem.

`a^2 + b^2 = c^2\\\\c = √(a^2 + b^2)\\\\x = √(8^2 + 8^2)\\\\x = √(2*8^2)\\\\x = √(8^2*2)\\\\x = √(8^2)*√(2)\\\\x = 8√(2)\\\\`

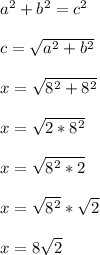
So that's why the diagonal BD is exactly
`8√(2)\\\\` units long

Side note:
`8√(2) \approx 11.3137`