Answer:
y = 2x+5
Explanation:
To find the equation in slope intercept form, we first have to find the slope of the two points.
The formula for finding the slope is:
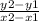
We are given the points:
(-1, 3) and (2, 9)
x1, y1 and x2, y2.
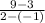
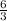 or 2 (the slope).
or 2 (the slope).
The slope intercept form is written as:
y= mx + b
y=2x+b
To find b, we can plug in either of the points given. Personally, I like to work with positive numbers. I'll be using the point (2, 9), however, either point will get you to the same answer.
y=2x+b
9=2(2)+b
9=4+b
Now subtract 4 from both sides.
5=b
Which then leaves us with the equation:
y = 2x+5