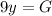Question

See ss below
spam answers will be reported​

Answer 1

`\boxed {\boxed {\sf G= 9y}}`

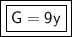
Explanation:

We are given the following equation and asked to find the missing factor that makes the equality true.

`18y^3=(G)(2y^2)`

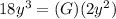
Essentially, we need to solve for the variable G.

1. Factoring

One method we can use is factoring.

We could factor the expression 18y³ because we know one factor is 2y². Since this is one of the factors, the other must be 9y.

`18y^3=(9y)(2y^2)`

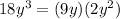
If we compare this factored version of the expression with the original equation we see that 9y and G correspond, so they must be equal.

`G=9y`

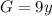
2. Solving

Another method we could use is solving.

We can solve the original equation for G by isolating the variable.

`18y^3= (G)(2y^2)`

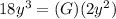
G is being multiplied by 2y³. The inverse of multiplication is division, so we divide both sides of the equation by 2y³.

`\frac {18y^3}{2y^2}=((G)(2y^2))/(2y^2)`

`\frac {18y^3}{2y^2}= G`

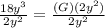
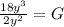
The coefficients are divided as usual and the exponents are subtracted.

`9y= G`

Answer 2

G = 9y

General Formulas and Concepts:

Algebra I

• Terms/Coefficients
• Factoring

Explanation:

Step 1: Define

Identify

18y² = G(2y²)

Step 2: Solve for G

Option 1: Factor

1. Factor: 18y² = (2y²)(9y)

Option 2: Isolate

1. Divide both sides by 2y² to isolate G: 18y³ / 2y² = G
2. Simplify: G = 9y