First let's compute dx/dt

Now compute dy/dt
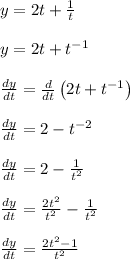
From here, apply the chain rule to say

We could use polynomial long division, or we could add 2 and subtract 2 from the numerator and do a bit of algebra like so
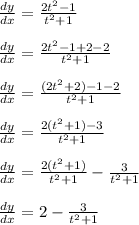
This concludes the first part of 4b
=======================================================
Now onto the second part.
Since t is nonzero, this means either t > 0 or t < 0.
If t > 0, then,
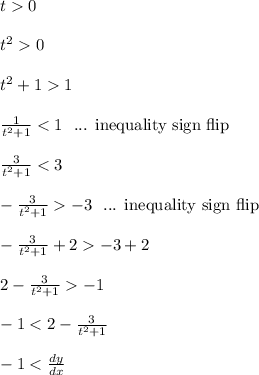
note the inequality signs flipping when we apply the reciprocal to both sides, and when we multiply both sides by a negative value.
You should find that the same conclusion happens when we consider t < 0. Why? Because t < 0 becomes t^2 > 0 after we square both sides. The steps are the same as shown above.
So both t > 0 and t < 0 lead to
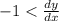
We can say that -1 is the lower bound of dy/dx. It never reaches -1 itself because t = 0 is not allowed.
We could say that
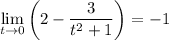
---------------------------------------
To establish the upper bound, we consider what happens when t approaches either infinity.
If t approaches positive infinity, then,
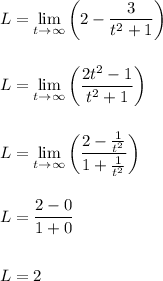
As t approaches infinity, the dy/dx value approaches L = 2 from below.
The same applies when t approaches negative infinity.
So we see that
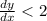
---------------------------------------
Since
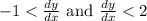 , those two inequalities combine into the compound inequality
, those two inequalities combine into the compound inequality
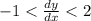
So dy/dx is bounded between -1 and 2, exclusive of either endpoint.