Answer:
The rate of change of the radius of the surface of the water when the radius of the surface of the water is 2 feet is approximately 0.637 feet per second.
Explanation:
The volume of the cone (
 ), in cubic feet, is defined by the following equation:
), in cubic feet, is defined by the following equation:
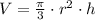 (1)
(1)
Where:
 - Radius, in feet.
- Radius, in feet.
 - Height, in feet.
- Height, in feet.
And there is the following ratio of the radius to the height is:
 (2)
(2)
By applying (2) in (1):

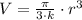 (3)
(3)
And the rate of change of the radius is found by differentiating on (3):
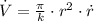 (4)
(4)
Where:
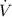 - Rate of change of the volume, in cubic feet per second.
- Rate of change of the volume, in cubic feet per second.
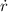 - Rate of change of the surface of the water, in feet per second.
- Rate of change of the surface of the water, in feet per second.
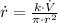
If we know that
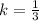 ,
,
 and
and
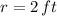 , then the rate of change of the radius of the surface of the water is:
, then the rate of change of the radius of the surface of the water is:
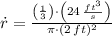

The rate of change of the radius of the surface of the water when the radius of the surface of the water is 2 feet is approximately 0.637 feet per second.