Final answer:
The graph of
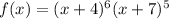 crosses the x-axis at the roots x = -4 and x = -7.
crosses the x-axis at the roots x = -4 and x = -7.
The answer is option ⇒ 1 and 2
Step-by-step explanation:
To find the roots of the equation
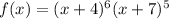 , we need to determine the values of x that make the function equal to zero and intersect the x-axis.
, we need to determine the values of x that make the function equal to zero and intersect the x-axis.
To do this, we set f(x) equal to zero and solve for x:
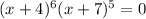
We know that a product of factors is equal to zero if and only if at least one of the factors is equal to zero.
Setting each factor equal to zero, we have:
x + 4 = 0 (Root 1)
x + 7 = 0 (Root 2)
Solving for x in each equation, we find:
Root 1: x = -4
Root 2: x = -7
Therefore, the graph of
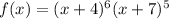 crosses the x-axis at the roots x = -4 and x = -7.
crosses the x-axis at the roots x = -4 and x = -7.
To summarize:
- The graph of
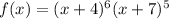 crosses the x-axis at the roots x = -4 and x = -7.
crosses the x-axis at the roots x = -4 and x = -7.
The answer is option ⇒ 1 and 2