Question

HHHEELPP HELP HELP!!
I need the answer ASAP!!!!

Answer 1

`\text{b. } y=-(x-3)^2+4`

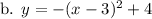
Explanation:

Algebraically, we want to compare the y-coordinates of the vertex, since all the functions shown are parabolas that are concave down.

Let's break the format down:

The negative sign in front of each of the functions indicate that the parabolas will be concave down (open downwards), which means the vertex represents the function's maximum. The term inside the parentheses when applicable to just indicates the horizontal/phase shift.

Since the first term being squared is negative, we want to minimize its value to produce the greatest possible y-value.

Therefore, substitute whatever value of
`x` that makes each
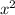`x^2` term equal to 0. (Maximum value of
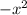`-x^2` is 0).

Therefore, we can simplify compare the last terms in each equation.

Equation A's last term is 3.

Equation B's last term is 4.

Equation C's last term is -5.

Equation D's last term is 0.

Since equation B has the greatest last term, it will have the greatest possible y-value.

Answer 2

Explanation:

B because the vertex is at point (3, 4) which is greatest.