Given:
The polynomial is:
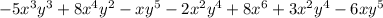
To find:
The simplified form of the given polynomial in standard form.
Solution:
We have,
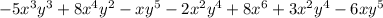
Combining the like terms, we get

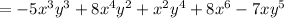
Now, rewrite this polynomial in standard form.
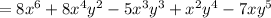
Therefore, the required polynomial in standard form is
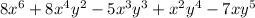 .
.