Given:
Diameter of the tennis ball = 14 inches.
Three tennis balls are fit in a cylindrical tennis ball cane.
To find:
The volume of air left between the balls and the cane.
Solution:
Diameter of the tennis ball = 14 inches.
Radius of the tennis ball = 7 inches
Radius of tennis ball is equal to radius of cane. So,
Radius of the cane = 7 inches
Height of cane is:
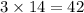 inches
inches
Volume of a ball is:
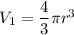
Where, r is the radius of the ball.
So, the volume of 3 ball is:
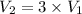

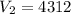
Volume of the cane is:
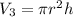
Where, r is the radius of the cane and h is the height of the cane.

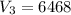
Now, the volume of air left between the balls and the cane is:
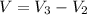
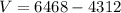
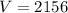
Therefore, the volume of air left between the balls and the cane is 2156 cubic inches.