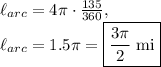Answer:

Explanation:
The circumference of a circle with radius
 is given by
is given by
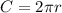 . The length of an arc is makes up part of this circumference, and is directly proportion to the central angle of the arc. Since there are 360 degrees in a circle, the length of an arc with central angle
. The length of an arc is makes up part of this circumference, and is directly proportion to the central angle of the arc. Since there are 360 degrees in a circle, the length of an arc with central angle
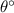 is equal to
is equal to
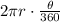 .
.
Formulas at a glance:
- Circumference of a circle with radius
 :
:
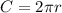
- Length of an arc with central angle
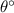 :
:
Question 1:
The radius of the circle is 12 m. Therefore, the circumference is:
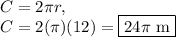
The measure of the central angle of the bolded arc is 270 degrees. Therefore, the measure of the bolded arc is equal to:
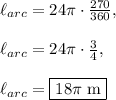
Question 2:
In the circle shown, the radius is marked as 2 miles. Substituting
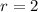 into our circumference formula, we get:
into our circumference formula, we get:

The measure of the central angle of the bolded arc is 135 degrees. Its length must then be: