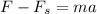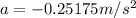Complete Question
A light spring with force constant 3.05 N/m is compressed by 7.80 cm as it is held between a 0.400-kg block on the left and a 0.800-kg block on the right, both resting on a horizontal surface. The spring exerts a force on each block, tending to push the blocks apart. The blocks are simultaneously released from rest. Find the acceleration with which each block starts to move, given that the coefficient of kinetic friction between each block and the surface is 0, 0.035, and 0.397. (Let the coordinate system be positive to the right and negative to the left. Indicate the direction with the sign of your answer. Assume that the coefficient of static friction is the same as the coefficient of kinetic friction. If the block does not move, enter 0.)
(a) u = 0 heavier block m/s2 m/s2 lighter block
(b)M = 0.035 heavier block m/s2 m/s2 lighter block
Answer:
a)
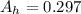
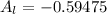
b)
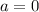
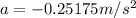
Step-by-step explanation:
From the question we are told that:
Force constant
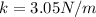
Compression Length
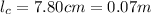
Left Mass
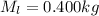
Right Mass
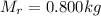
Coefficient of kinetic friction
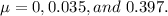
Therefore
Spring force is given as

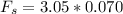

Generally the equation for Acceleration is mathematically given by
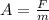
For Heavier block
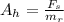
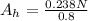
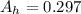
For Lighter blocks


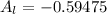
b)
Generally the equation for Force is mathematically given by
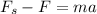
For Heavier block
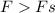
Therefore
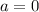
For Lighter blocks