Solution :
We observe that :
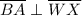
But BA is the perpendicular.
From the center B and WX is a chord.
Therefore, TW = TX (perpendicular from the centre of a circle to a chord bisects it)
Consider Δ BTX,
∠BTX = 90° (BA ⊥ WX)
BT = XT (Δ BTX is isosceles)
Since the angles opposite to equal sides are equal of a triangle arc are equal.
∠BTX = ∠BXT
But in the triangle,
∠TBX + ∠TXB + ∠BTX = 180°
∠TBX + ∠TBX + 90° = 180°
2 ∠TBX = 90°
∠TBX = 45°
From trigonometry, we get
 ...............(1)
...............(1)
WX = 10
i.e., TX + TW = 10
But TX = TW
2 TX = 10
Tx = 5
BX = radius of circle.
∴
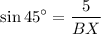

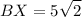
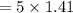
= 7
Therefore, the radius of the circle is 7 units.