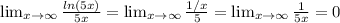Answer:
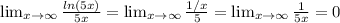
Explanation:
L'Hopital's rule says that, if both numerator and denominator diverge, then we can look at the limit of the derivates.
Here we have:
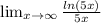
The numerator is ln(5x) and when x tends to infinity, this goes to infinity
the denominator is 5x, and when x tends to infinity, this goes to inifinity
So both numerator and denominator diverge to infinity when x tends to infinity.
Then we can use L'Hopithal's rule.
The numerator is:
f(x) = Ln(5x)
then:
f'(x) = df(x)/dx = 1/x
and the denominator is:
g(x) = 5*x
then:
g'(x) = 5
So, if we use L'Hopithal's rule we get: