Question

How can an expression written in either radical form or rational exponent form be rewritten to fit the other form? Explain thoroughly and thank you for your time.

Answer 1

When you're given a problem in radical form, you may have an easier time if you You can rewrite every radical as an exponent by using the following property — the top ... For example, 643/2 is easier if you write it as (641/2)3 = 83 = 512 rather than (643)1/2, Rewrite the entire expression using rational exponents.

Answer 2

Explanation:

We'll start with going form exponential to radical, since it may be easier to follow. For example,

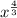`x^{(4)/(3)` can be written, in radical form, as

`\sqrt[3]{x^4}`. What happens is that the denominator of the fraction serves as the index (the number that sits in the bend of the radical) and the numerator serves as the power on the variable (or number, if that's the case). Let's look at another one:

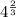`4^{(2)/(3)` becomes

`\sqrt[3]{4^2}=\sqrt[3]{16}=\sqrt[3]{8*2}=2\sqrt[3]2}`

And you can go the other way with it. For example,

`\sqrt[5]{x^4}` becomes

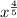`x^{(4)/(5)`, etc. Get it?