Question

Use the normal distribution and the given sample results to complete the test of the given hypotheses. Assume the results come from a random sample and use a 5% significance level.

Test H0 : p=0.2 vs Ha : p≠0.2 using the sample results p^=0.27 with n=1003
Round your answer for the test statistic to two decimal places, and your answer for the p-value to three decimal places.

Answer 1

The value of teh test statistic is
`z = 5.54`

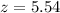
The p-value of the test is 0 < 0.05, which means that there is significant evidence to conclude that the proportion differs from 0.2.

Explanation:

The test statistic is:

`z = (X - \mu)/((\sigma)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

0.2 is tested at the null hypothesis:

This means that
`\mu = 0.2, \sigma = √(0.2*0.8) = 0.4`

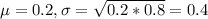
Using the sample results p^=0.27 with n=1003

This means that
`X = 0.27, n = 1003`

Value of the test statistic:

`z = (X - \mu)/((\sigma)/(√(n)))`

`z = (0.27 - 0.2)/((0.4)/(√(1003)))`

`z = 5.54`

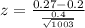
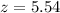
P-value of the test and decision:

The p-value of the test is the probability that the sample proportion differs from 0.2 by at least 0.07, which is P(|z| > 5.54), that is, 2 multiplied by the p-value of z = -5.54.

Looking at the z-table, z = -5.54 has a p-value of 0.

2*0 = 0.

The p-value of the test is 0 < 0.05, which means that there is significant evidence to conclude that the proportion differs from 0.2.