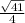Answer:
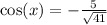
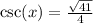
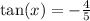
Explanation:
We know that (-5,4) is the terminal side. This means out legs will measure 5 and 4 if we graph it on a triangle.
We need to find the cos, csc, and tan measure of this point.
We can find cos by using the formula of

The adjacent side is -5 and we can find the hypotenuse by doing pythagorean theorem.
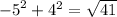
So using the info the answer is
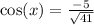
We can find tan but first me must find sin x.
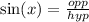
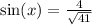
So now we just use this identity,
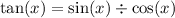
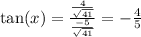
So tan x=
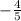
We can find csc by taking the reciprocal of sin so the answer is easy which is